Research into VBM, Fibonacci and Lucas Numbers
(Inspired by a discussion on the VBM Work group forum (vbm369.ning.com/forum/topics/fibonexus-1) exploring the relationship between the Fibonacci sequence and Marko Rodin’s Mod9 “nexus key)
First let us look at the mod9 nexus keys. If you examine Rodin’s mod9 number maps (see mod9 ANALYSIS.pdf) you will understand that there are three.
Rather than looking at the 124875, and 933966 sequences, look at the sequences perpendicular to these. These 18 digit repeating sequences are called the nexus keys. There is one for each map:
[18]: Sequence 165297438834792561
[27]: Sequence 231495867768594132
[45]: Sequence 173553719816446289
So how do we find these within the Fibonacci sequence, and what about other moduli?
As members of the forum pointed out, Fibonacci numbers can be divided into smaller Fibonacci numbers and whole integer results can be found at regular intervals in the sequence. For example every seventh Fib number will divide into Fibonacci number 13. I charted all the results of these divisions, and reduced the results to mod9, mod25, and mod49. Once repeating patterns were found for each moduli, no higher Fibonacci / Lucas numbers were explored.
Data for mod9
Fibonacci divided into Fibonacci numbers reduced to modulus 9 are shown in the first file:
fib divided by fib mod9 DATA.pdf
Quite simply every fib number is tabled down the vertical axis of the table. The divisors are arranged across the top axis. Every number that will divide into a Fibonacci number and remain whole is shown – reduced to mod9.
I noticed every sequence for each divisor, going down through the table is very specific and has a repeating pattern.
I created a second set of data:
Lucas divided by lucas mod9 DATA.pdf
SUMMARY OF ALL mod9 DATA
I summarised the (repeated) sequences of both sets in the third file:
This allows us to see more clearly the specific mod9 sequence that applies to each divisor for both Fib and Lucas. There are many interesting things about these groups of sequences, manly noted below the table. However for now the key thing is this: Notice that the 18 digit sequences are all the same in the Fibonacci group, are attributed every 8th sequence beginning with sequence 4, and are all identical. They form the Rodin Nexus Key for one of the 3 Rodin number maps – to be found at the end of the document. So where could I find the other two nexus keys that applied to the [81] and the [27] Rodin maps?
For the Lucas group there is an interesting pattern to the sequences, noted below the table. Here the nexus key occurs with greater frequency: Every 4th sequence beginning with sequence 2 forms the 18 digit Rodin Nexus Key that makes up the [81] Rodin number map
Making the [27] Rodin Map is then fairly easily deduced from this data as shown in the notes.
Data for mod25 and mod49
I have found the Nexus keys and therefore number maps for each of these.
Mod25
Fib divided into fib mod25 DATA
Mod49
fib divided into fib mod 49 DATA
lucas divided by lucas mod49 DATA
Mod 16 - not a prime squared - therefore will not create maps
I checked Mod16 to see if there was anyway to achieve a number map with this. It is not possible.
Sorry I lost the fib divided by fib mod16 DATA
lucas divided by lucas mod16 DATA
Mod81
It would be a good to follow up to check if mod81 works. To follow Randy Powells theory, it should not, and the next workable number maps should be derived from a mod121 analysis. I did this research in a very slow way - so doing the reseach for mod81 or mod121 would be too laborious. If someone would like to automate it and come up with the data to include here please do - all credit will be given.
Regarding copyright - please ask before publishing elsewhere. I generally am open to it to be used by others, but please credit me, and include links to this webpage.
Other calculations that led to "Phi VBM Tori Array" film
The document below is not 'rigorous' mathematics - apologies in advance to anyone who expected this. It's my own way of working. Any constructive thoughts, feedback, or additions are welcome.
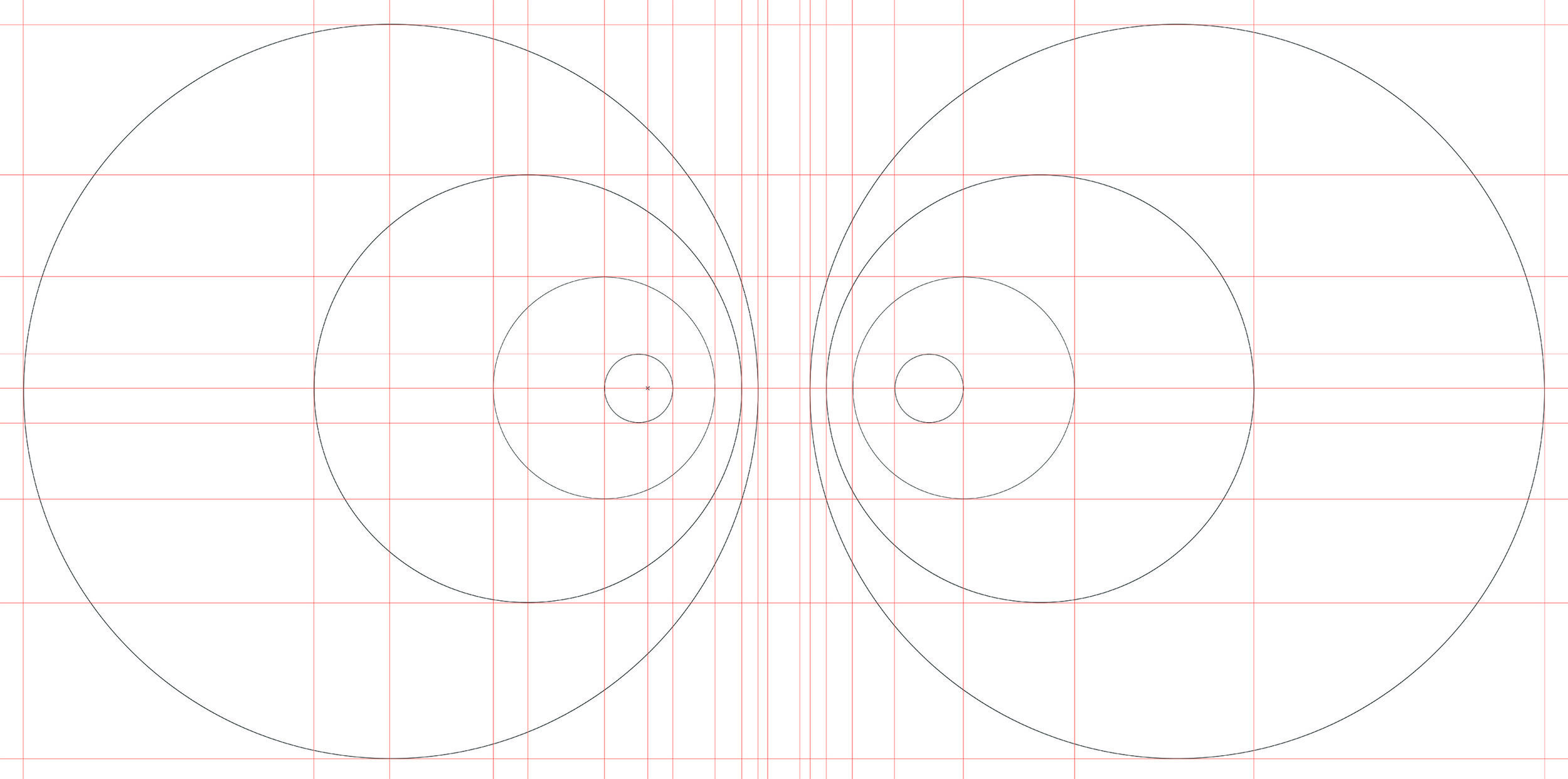
Phi VBM Tori Array Relative Proportions Diagram
A cross-section of the first four tori, showing the onion-skin effect. The 9x36 is defined by the smallest circles, next circles up 36x36, next up 99x36, next 261 x 36. It continues infinitely.
Phi proportions can be seen everywhere by comparing distances between straight lines.